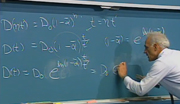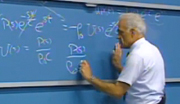Amar G. Bose: 6.312 Lecture 12

DR. AMAR G. BOSE: Last time, we did acoustical mass, which was an open tube. We did acoustical compliance, which was a closed tube. We derived these both two ways each, one from the wave equations and two using information we got from the wave equations. In the case of the acoustical mass, which was the open tube, looking at the standing waves we found out that the velocity essentially was constant over the whole length. And if the velocity is constant, then the impedance you see in here is as a result of moving the mechanical mass of air, which moves just like a slug in there. And when we just used Newton's law on that, we got the same result.

In the case of the compliance, we realized from the single tube that if the wavelength is large compared to a device, it doesn't matter which way the waves are really going when they hit a surface. It generates-- of course, the boundary condition is velocity 0, so pressure doubles, and it basically sends a wave back. But since the distances of this strange object are all small compared to 1/6 of a wavelength, the pressure is constant everywhere in there. Realizing that, we went to the gas law and developed the same expression for impedance exactly, because that gas law really assumed that the pressure was the same all over.

That left us with just one element to worry about. And that's the resistance element. We really haven't brought in loss up until this point. Loss, as I mentioned earlier, is in acoustics due to friction. And anything that you can think of that will cause friction between the molecules of the air and some body as the air moves will cause loss. Loss converts acoustical energy into heat.

So there are various forms of devices, if you wish, that cause loss. A very thin tube will cause loss, not by any mechanism that we have already looked at but simply through the fact that air has viscosity. And so if you have air flowing through a tube, the air in the center will be going at a higher velocity, just like your fluids, than the air at the end or the edges of the tube. If you looked at the edges of the tube under a microscope, they would look like mountains. What looks to us like it a smooth surface inside the tube it would look very, very ridged, and there's no velocity to speak of at the edges, but there's a big velocity, or some velocity, at the center. And the velocity decreases as you go out. And when you have layers of air that are going faster than the ones adjacent to them, that causes viscous friction.

So that's a loss that is within the medium itself. Yes, the medium does intersect something at the edge of the tube, but all the way through the tube, the loss is just because of the viscosity of air, which is something like 10 to the minus fifth, or 1.8 times 10 to the minus fifth newtons means per meter squared seconds, I think viscosity is.

You may remember from physics, you had a coefficient of viscosity, and it was related like this. Pressure is this so-called viscosity times the spatial rate of change of the velocity. Pressure is this times dy dx times the velocity, or times u, I'll put down. This just means that if you have a bigger-- y is the dimension up here, x is the dimension over here. If you have a tube coming along here, you have a gradient in velocity. If I were to draw the velocity-- let's say I was pushing air through here. It's 0 at this point. It's a little bit bigger here, bigger here, bigger here, bigger in the center, and et cetera. A similar distribution going down. It's the spatial rate of change, if you wish, that causes this. So the more viscous the fluid is, the bigger eta and the more loss you would have for a given velocity.

So the interesting thing about all these loss forms is-- and this is fundamental. It's a root of it, and we'll see in a minute why if architectural acousticians don't understand this, they will wind up with very funny surfaces that aren't doing anything and are very expensive. This whole business of changing acoustical energy into heat needs velocity. Velocity gradients, or if you're sending an acoustic wave through a material, if you do it at really low frequencies, one cycle per day, oh, heck, there's nothing converted to heat. But just like that shock absorber, if you try to force it at a higher velocity, then you convert a lot to heat. We'll get some examples of this, even in one dimension, that will show us how to use acoustical materials in a few minutes that are really applicable to three dimensions. And we'll see it again when we go to rooms.

A few types of acoustical materials that make up loss. Thin tubes, because if you have a wide tube, your velocity profile would be like this, let's say. And then it gets out here, and then there's not much change anymore. The real change takes place as you get near the edges, where clearly, if the tube were as high as this house, and the waves going through, all the loss would be at the edges of the tube, and nothing would be in the center because these velocities would be essentially now about the same. So narrow tubes are one form of resistances.

Porous materials, sintered materials, with any kind of material in which the air going through has a maximum contact with the surfaces of the material as it passes through. Narrow slits, window screens. These things comprise essentially what we know as resistances today.

Now let's take a look at just one. Most of the values of these resistances are done empirically, just because it's pretty complex to compute them in real situations. And so they measure them. But some you can compute. Here's one. I'll just show you the result of this. I think I want to do this another way. I'll put it here. I have a plate and I have another plate. These would be pretty close to each other, in fact, normally. Bottom plate. Let's say that the wave is coming in like this. They have a width w. They're broadside to the wave. A thickness, t. And a length along the distance of the wave, L. What happens is if you write the acoustic impedance, the sub a for acoustic, if you wish-- acoustic impedance, which is defined as pressure over volume velocity-- you get an expression like this. 12 eta-- the viscosity coefficient, eta-- L over t cubed w plus j times 4/3 m-- mechanical mass-- times omega.

Now just look at this expression. The only thing I'm interested in is interpreting that expression here. This part is a resistance. But look what came along with it. This is a mass. In fact, if there were no resistance, this term would just be j omega times mechanical mass. So what happens so often-- when you're trying to get a resistance, you get a resistance and a reactive term too, because the resistance involves a velocity travel. And the velocity is what gave us mass in the first place. So actually, if you take pressure as the across variable and you make that analogous to voltage, you'd come up with a circuit like this for the impedance, in which voltage was the across variable. So you'd have this thing being 4/3 mm, and the resistance being everything that's over here.

Now clearly you can do some things to play around here to get what you want. I mean, if you only want a resistance, make t, the spacing between the plates, very, very small, so you get the maximum gradient all the time. I didn't draw it here, but if they're very, very small, the gradient is enormous and you don't have any region where there's no gradient. So making t small would cause this fellow-- i.e. this one-- to be big relative to this. And of course making t small reduces the mass, also, that you have in there.

The mechanical mass-- the reason I've written it in this form is mechanical mass is just the rho 0 times the volume. So you take the two plates and you squeeze them down. The volume goes down. So by making t small, you make this fellow small, make this one big, and you tend to get closer to a pure resistance, or at least you don't have to worry about it until you get to real high frequencies where the inductor dominates.

The best pure resistance we have is a window screen. Why? Because there's a lot of friction as you go through this thing, and there's no distance to travel, no acoustic mass. So that's about the best pure resistor, and in this sense, I'll refer you to the-- he has nice tables to show you what values there are. If you take a window screen and you increase the number of whatever-- if it's a cloth, the number of threads per square inch, or if it's metal, you bring them closer and closer-- you get a bigger resistance, as you would expect, where I define resistance with the across variable as being pressure, pressure over velocity-- you make these things smaller. If space is smaller, you get a higher resistance and negligible mass.

You can buy these materials, by the way, according to their resistance, just like you buy resistors off the shelf. You can buy cloth which has the right density for a given resistance. So that gives you this elements that's in here. And let's see, let me talk a little bit about the use of such a thing. This will become very important when you get to rooms like this, but you can get the whole essence out of it by looking at a simple situation.

Suppose I have a closed tube here. And just to make it simple, I'm going to put a fixed frequency, omega, in here. I'll run down a plane wave. And I have a sheet of this cloth, and I want that sheet to do the best job of absorbing energy that it can. Where will I put it? What orientation and where will I put it in there? Yeah?

SPEAKER 2: [INAUDIBLE] velocity maximum or the envelope [INAUDIBLE].

DR. AMAR G. BOSE: Velocity-- if I drew the standing waves for velocity, it's sine because they're 0 at the origin. The envelope looks like this. So what I would do, if I wanted to absorb that frequency to the most, I'd exactly do that. This is the velocity waveform. I'd stick the cloth here. If I stuck the cloth over here, it doesn't do anything, essentially. I mean, yeah, the wave bumps into it and you might say that the impedance it sees isn't quite infinite here. There's a little tiny bit of loss, you can imagine, in that cloth, but there's no velocity going through it. And so there's essentially nothing. And so the same piece of cloth placed out here will do a lot.

So what this tells you-- in the hall, for example, people will say, oh, if there are too many reflections in the room, but you have to do is put absorbing material there. And the more dense that material is, the more friction there is when the wave goes through it. Well, you can take a very dense curtain and put it right here along the wall and it won't do a fraction of what it would do if you put it out here, because there's more velocity as you go. And now we're not talking one frequency. I used that just for illustration. But the waves in the room, as you'll see, are pretty complex. And in general, you'll have a heck of a lot more velocity out here then you'll have here.

So if you hung that curtain out here, you'd do an enormously effective job. And you can do it either by making the curtain dense or you can just use a lot of pleats, run it along here. But put the same thing over here against the wall, and it won't do it. So if you don't understand the principles, you can see what will happen. People will just think, oh, a curtain is a curtain. It absorbs energy. Put it in the walls, it takes up less space. It takes up less absorption, too.

OK. So that's about all I want to say at the moment for resistive elements. And I'll say only a little bit more for transformers. Transformers in electrical engineering-- the have obvious use in mechanical engineering. They're common as models of things like levers and gears. In acoustics-- at least this is my feeling. There may be some people in other aspects of acoustics that have a different view. But I've always looked at these transformers in acoustics as something in which you force the real world into the structure of this thing. And that's very bad. I mean, the transformers should be something that is useful to approximate the real world. You shouldn't force the real world into a model that you have.

There aren't any that I know of, transformers in acoustics, that are approximating as well as you get then the model and the physical world in mechanical engineering or electrical engineering. And there's some wild approximations, if you happen to look at one of these texts like Beranek, for example, where he takes the essence of papers and the results and puts them in there-- there are approximations to get these transformers that are A, very complicated, and B, you wonder if it's worth it in the end, what you get.

But here is a transformer that's often looked at in acoustics. You'll see it in acoustics texts, for example. You have an area of this tube, A1, and an area of this tube, A2. A pressure 1 here, a volume velocity 1 here, a pressure 2 here, a volume velocity 2 here. OK. Anybody have any idea what the boundary conditions are right here? I'll say that that's x equals 0. What do you think the boundary is on pressure between the two right here? If you had to guess, what would you say?

SPEAKER 3: Pressure on one side is equal to pressure on the other side?

DR. AMAR G. BOSE: Yeah. [INAUDIBLE]. Something's taking [INAUDIBLE] right? So the assumption [INAUDIBLE] pressure 1 equals pressure 2. How about right at this point here, the volume velocities? What would you say about volume velocities, if you were forced to guess at that without calculations?

SPEAKER 4: V2 equals A1 over E2?

DR. AMAR G. BOSE: Let's see. The volume velocity is the volume that's moving across that surface. In other words, I'm right here, right at the entrance of this. What would you say?

SPEAKER 5: [INAUDIBLE].

DR. AMAR G. BOSE: The answer I want is the wrong answer anyway. [INAUDIBLE].

SPEAKER 6: Same on both sides?

DR. AMAR G. BOSE: Same. Now why? Well, it's very hard to explain why for something that isn't right. But you get the feeling, well, hey, if there's is a certain volume of air right here, it's got to be moving across here. Now if you use these approximations, you get a transformer like this. Two variables-- VV1, VV2, P1, P2. What do you think the transformer ratio is? Big deal. 1 to 1, right? Not too exciting a device.

Now the actual problem. You see, if the second area of the tube was very, very small, a little straw coming out of here, you would say, hey, wait a minute, I know that the wave is going to come in, hit this wall-- it can hardly see the little hole-- and another P plus is going to go back just like it was a closed tube. If this area 2 were very close to the area 1, you'd say, I know that this is really a continuation of the tube.

Well, what happens is this is not a trivial problem to solve. In the days when it was first solved in closed form, it's a formidable problem. Today you'd use finite element analysis and the boundary conditions, and you'd actually plot it out on the computer. But all sorts of reflections occur in this area here, where it does hit something. And remember, when sound hits a rigid surface, something reflects. And so in this area, the field is not at all a plane wave. And so these approximations are interesting, but you have to be careful if you want to use them.

And I guess this is a dangerous thing to say, because I don't want to preclude applications for you, but I've never come across a situation where I needed to use anything like this. And I would be very skeptical if I did, unless it was one of the near extremes. If it was a very small tube here, then I would say, aha, I know the pressure all over here, and the wavelength is very large compared to that, so the pressure is going to be about the same right at the tube. And then I could begin to work from that, to say that's the pressure that will launch a wave down this narrow tube. Things like that, I wouldn't be too unhappy to use. Much more, I would.

It's interesting to take a look at this transformer, though, and say, suppose I were using this transformer not as an acoustical transformer, but for the same physical structure-- let's suppose this is all minute compared to 1/6 of a wavelength. Let's suppose I drove it here with some velocity source or some source-- piston-- and this is all very small. And maybe at the other end of this, I had some sort of a diaphragm, and I drove a mechanical system of masses and dashpots or whatever it was, so that I'm interested in this thing as a mechanical transformer, in which the variables are velocity 1, force 1, velocity 2, force 2.

Now since P1 was equal to P2, well, P1 is F1 over A1. And that's P1. And P2 is equal to F2 over A2. P2. Now volume velocities were equal, so VV1 is equal to U1 times A1. And that is equal to VV2. This is P2, in case you wondered. VV2, which is U2 times A2. So I took those boundary conditions, and I just turned them into mechanical variables. So now I have a transformer in which, from this equation, F1 is equal to A1 over A2 times F2. From this equation, U1 is equal to A2 over A1 times U2. So all of a sudden now, my cross variable is here, F, and so this is a transformer, A1 to A2, or said in another way, A1 over A2 to 1. It's a transformer of the type we're used to seeing.

So when you go from one discipline to the other, you can see that interesting things that you might not have thought would happen. This was sort of an academically interesting thing. And all of a sudden, you've got a transformer here which is real. It's the kind of thing that you think about that's nontrivial.

Now we mentioned that it's the reflections from surfaces like this that cause trouble. You do want to change impedances sometimes. In electrical, it's quite common. For example, in the early days of television, it wasn't so simple to make. The magnetic materials weren't good. It wasn't so simple to make a transformer which would take your, let's say, 75-ohm input of a coax and get it to a 300-ohm input on the TV. And you needed to make that impedance transformation. So they had same problem we do, because everything in electrical is derived from waves.

And what they would do is they would say, well, look, if I have a transmission line that has this spacing, let's say, between the two wires, and I would like one with a different impedance, which would have a different spacing between two wires, what I ought to do is make this thing very long, and sort of creep from there to here. So to just go like that, maybe.

And then the question becomes, if you want to do that, how do you creep? Do you do it with straight lines? Do you do it with parabolas? What do you do? What's your guess? Anybody want to guess? In other words, I'm looking for the shape of this thing here. OK, now I'm going to tell you. You're all engineers. Give an engineering guess. When in doubt-- oh, boy.

SPEAKER 7: Maybe like a half a sine wave or something?

DR. AMAR G. BOSE: Half a sine wave? No.

SPEAKER 7: Exponential.

DR. AMAR G. BOSE: Exponential. When you're an engineer and you're asked anything, when you don't know anything about it, start with an exponential [INAUDIBLE]. The probability is very good. Now there's a very interesting reason why the exponential is the shape that you want to get. You see, it's the change in area as you go from one section to the next that causes reflections. If you have any other shape, well, let me just say that the area of this thing as it's going out here-- if I call this x and this 0, let's say, that the cross-sectional area in here, A, is going as an exponential, which means the radius is going in as an exponential. So let's say the exponential is e to bx.

Now dA dx equals b times e to the bx, is equal to b times the area. Or dA over A equals b dx. So that if you move a distance, dx, you get the same percentage change in area at any point here. In other words, if I get 1/10 of a percent change in area by moving a little dx here, the same motion, the same amount of dx, gives me 1/10 of a percent change in area over here. Any other shape-- for example, suppose I did a linear one. This will have a much bigger change right here, than it has here, because in moving a given distance, dx, here, when the neck is very small, you move the same amount vertically that you do out there, but the percentage change is much greater over here.

Now that is a very nice argument, once you've seen the whole solution, but just to tighten the thinking process-- I mean, it sounds very good and it's correct, but if you hadn't done the analysis of this, you might ask, yeah, I know, but this has a big change right here, but when you get out here, it has less change than the exponential. And so you have a large region with less change and a small region with a big change. How do I know that they don't average out and that it's not all the same.

So you could not approach a problem the way I just did the first time with that kind of an argument, without checking yourself and saying, yeah, but that sounds good, but so what? I only have this large region here and abut a small region out here. I don't know. You'd have to really work it out. But it does turn out that way, that it's the smaller region, the region where you have the biggest dA dx, that dominates and causes standing ways to go back.

Now the effectiveness of transformers like this-- by the way, this was actually used in television sets in the '50s, for example. If you looked where the antenna connected, you'd find a twin lead which started out at about a separation like that, and went to maybe a separation like that, that got the 75 to 300 ohms. And what they would do-- they didn't have too much space for it. There was in those sets, because they were big, but they would the twin lead up, and it still worked pretty well. In other words, you'd take these wires that went like that, and then just roll them all up and package them in there, and you'd see that lump inside, if you looked behind the antenna connector.

A way that is often used in any discipline with waves to measure the effectiveness of this thing is to measure the standing wave ratio here, because if this is terminated properly at the other end, in whatever impedance there is, and this were perfect, there'd be no standing wave here, because there would be no reflection. So the better the device, the lower the standing wave ratio is at this point.

So that's the way you can measure it. I mean, that's even the way you measure the effect of how good a connector is. You can go to Radio Shack or these places, you buy the cables with connectors on them that connect your antenna to the jack on the wall or something. Well, the connectors are actually rated. Not in a place like that, but if you had bought them for more technical use, the connectors are rated for their standing wave ratio. So you take a line that's terminated somewhere here, and you put a connector here to that line. the line comes in here, the connector's here, this is terminated, and then you measure the standing wave ratio here. And the connectors, the price goes up with the inverse of the standing wave ratio.

Now another reason why these things aren't really transformers is if you want to model this as a transformer, you can do it. You model it as a transformer plus a delay, because it takes time for the signal to go from here to here. And there's energy storage in that. Remember, our transformer-- if you multiply the F times the U here, that's instantaneous. The power going in is the power coming out. Power going in here is not power coming out here, because there's a big area and there's a lot of energy in there and it stores. So a first-order model would be a transformer cascaded with the delay unit.

OK. The resistance element, by the way-- you have to have a symbol for the thing. It's like a screen, like looking at the cross-section of a screen is often used. If you have a tube in there, and you have this kind of a symbol across it, that's just what's commonly used for resistance.

Now we have the elements--

SPEAKER 8: Professor Bose?

DR. AMAR G. BOSE: Yes.

SPEAKER 8: Going back to the screen placement, in an anechoic chamber when you have wedges on the wall, the distance the wedge sticks out from the wall determines what frequency range it will attenuate?

DR. AMAR G. BOSE: Yeah. The question was asked about an anechoic chamber. You'll see one of these on the last day, if you haven't seen them. But they have big wedges like this of fiberglass. All fiberglass, sometimes held together with a fine wire mesh which hopefully doesn't give too much reflection. The object of an anechoic chamber-- anechoic, meaning no echoes-- is that sound will hit the wall and not come back. And so these wages are adjacent to each other. So the next one goes down like so. And the key is the bigger they are, the longer the wavelengths that they will attenuate, the more attenuation you can get.

Everything gives a reflection. The more gradual it is, the wave sneaks in between here and starts to have just a little loss here, a little bit more loss here, a little bit more loss as it goes out. If you didn't do this and you packed the fiberglass just smack against the wall, what would happen is you would most certainly get a reflection from that. And so this is a way of getting the wave in with minimum reflection, so to speak. And that's why they point the thing. And yes, the bigger they are, the lower the frequencies you're going to get.

OK. Let's see. What was I going to do? Resistance, transformers-- yes. Well, let's see. It looks like we have to do KVL and KCL before we can do circuits, because all circuits are elements, by definition, connected to each other. So the laws of connection.

Not so different than the mechanical engineering. If you have a volume velocity 1 here, a volume velocity 2 here, a volume velocity 3 here, clearly you can't pile up the volume here, the material and the particles that are coming in. And so you have Kirchhoff's Current Law for this situation. And that's why it's sort of natural, for an EE at least, to think of the final velocity as the through variable. Whenever I have to make an acoustical circuit, my thinking is always through variable is volume velocity. And I come up with the circuit.

It's not going to take too long-- in two or three weeks, you'll see very often we want velocity as the across variable. So fine, get it the way it's easy to write, take the dual, and you have it. So whichever discipline, whichever set of variables you want to take as the across variable and through variable, if it makes it easy for you to write it, write it that way, and if you need it in the other one, take the dual.

So KCL would say summation at the node of this, so of the VVj's, is 0, where that symbol means same thing we do in electrical and mechanical, namely that it means that you can sum the things going out or coming in, obviously. 0 is minus 0.

So this, in this case, would be-- if I do it going in-- VV1 minus VV2 minus VV3 is 0, which is this sum. Then we also have a KVL for acoustical circuits. You can assign a pressure all around this thing in space. And the sum of the pressure drops across the elements must bring you back to the same pressure. So the KVL is summation around the closed loop of pressure drops, is equal to 0. Whatever kind of a situation you have, you just go around the whole model. It could be anything. Summation of the pressure drops. This is a pressure rise, if you're going clockwise. Pressure drop. Summation must be equal to 0. That enables us to have a network theory, if you wish, for acoustical circuits. And now we can actually go to them and see some interesting results.

Let's suppose I just take a normal-- some of the kind of things you'll see in living rooms of fancy houses, where they collect different items, you'll see a giant thing that may sit this high, a big vase of some sort, decorative. There it sits in the living room. This has a volume, V0, let's say. This has a length, L. This has an A to it. What do you think the equivalent circuit is, looking in here? z acoustic impedance, which is defined as pressure over volume velocity. Let's make the analogy that pressure-- see if we can go all the way-- well, no, it won't. Give me the acoustic elements in the acoustic circuit for this. I will tell you-- just one second. Did we give a handout on the termination of tubes? Today. OK. Pick it up. Let me see it. OK. Thanks.

What you need to derive-- I spoke to this a little bit last time when we got to the open tube. This is on the side here. Remember, we said we derive this open tube as an open tube, and for the impedance only in here. One port. A port is an entrance to a network with two terminals. The terminals here aren't exactly obvious, but it's two variables, the volume velocity and the pressure you have across here. Pressure and volume velocity.

But then we said, aha, on certain circumstances, if we put things on the end of this, we can still look in here. The certain circumstances we derived for you in there, and I can tell you basically what they are-- if this is z0 in here, that the impedance magnitude that you see out here is very, very small. The zL is very, very small compared to z0, because the assumption we made in deriving this was that the pressure was 0.

As we're going to find out next week, the pressure isn't quite 0, because it sees the world out here, and when the velocity comes to the end, the world generate some pressure at this point. But because it's pretty big out there, the velocity can go all directions, and the pressure that it generates isn't very big. And so we made the assumption P was 0 out here to derive the result that this was an inductor of value rho 0 L over A, where this through variable is volume velocity, and the cross-variable was pressure.

Now what we need to show, and that will be shown, is that when you put something on here-- and I don't know what kind of symbols to use for that, but I'll put something on here which, looking in here, zL-- that when that zL is very small compared to z0, what happens when you look at the impedance in here is that you get the impedance of this plus the impedance that you see looking in here. That's not obvious. But as you'll see, it does come right out of the expressions, under the approximation that zL is very, very small compared to z0. So the through variable is volume velocity.

I'll try to just make it make sense to you from a physical point of view. When the pressure is very, very low here, you get the same standing wave in here is if it were 0. And the standing wave in here says that the velocity-- this is very small-- is essentially constant. It goes as a sine wave, but it doesn't get down until way out there. So velocity is essentially constant, or volume velocity because the area is fixed. Volume velocity here is essentially volume velocity here. That's going into this load that generates a little bit of pressure here, a tiny bit.

And the interesting thing is when you look at what the pressure is out here, it turns out to be whatever it would have been for an open tube, plus the pressure that's generated by that volume velocity, which is constant through there going into this fellow. So pressure here is pressure here, plus this. And that of course is exactly what would happen if you had two elements that were zL and this mass, rho 0 L over A, in series. Volume velocity, VV, is common to both, so they're in series. That's what tells you whether elements are in series, through variable common. If cross variable is common, then they're in parallel. So you do find that this is a model for a tube terminated such that zL is much much, much less than z0. So we need that on the side.

Now I will tell you that this jug that's sitting in someone's living room here, this big part, the base of it, is large enough to satisfy those inequalities. Now what I need is the equivalent circuit for this, looking in the terminal pair that's here. Come on. I'm not going to do this. I mean, I am not. You are. Here's the terminal pair. I'll do that much for you. Yeah.

SPEAKER 9: [INAUDIBLE]?

DR. AMAR G. BOSE: Same as I've drawn here? What's this?

SPEAKER 9: This is the impedance of the v0, the big base. [INAUDIBLE].

DR. AMAR G. BOSE: Rho 0 L over A. And the impedance of that is, as we know from last time, V0 over rho 0 c squared. That's the impedance.

Now I'm going to go a little bit ahead here. We haven't talked too much about fields, and we haven't talked at all about three dimensions. But there's some very practical consequences of those things. That vase, if you want to call it that-- this thing's sitting in the living room, this decorative thing. If you put a hi-fi system next to it, or if you sat down next to it and you talked, you would sound quite a bit different, believe it or not.

At resonance frequency, what's the pressure at this point? In other words, let's suppose the room was excited by somebody speaking, or by music, and at resonant frequency of this and this, what's the pressure here? 0, sure. Pressure is the across variable, like voltage. Volume velocity, the through variable. The impedance of this thing goes to 0 at resonance frequency. So the pressure here is 0.

Now if that frequency is such that the wavelength is reasonably large-- in other words, this is a big jug now-- then the pressure isn't going to be 0 here and some big number over here. That 0 is going to eventually die out, but it's going to be, for a surrounding area, appreciable. So that can actually affect the sound in a room, that jug.

There is a very interesting way that used to exist-- it still does exist. Today, though, you would do it differently a little bit, or many places would. You can map the sound pressures in a room. You turn on the source at any frequency, put it anywhere you want, and then you'd take a microphone-- and usually this is automated-- and the microphone would have different color little bulbs on it, normally diodes, and the circuitry that the microphone is connected to would light, let's say, the green light when the sound pressure level was 80 dB. When it's 90 dB, it'll light the yellow light, whatever. It goes on up.

Now you turn out the lights. You open a camera back here, and you move this thing, maybe mechanically, through the room. And you get, then, a photograph of the lights that are lit, and you can see what the sound pressure level is all over the room. And that was used, for example-- you have a door here, you make a noise outside, and you want to find out where is that noise coming into the room? Well, is it the wall that's vibrating? Is it leaking through the door? And you when you get near the door, you see these contours, and you see exactly where the high sound pressure level is and it's coming through.

Today, if you want to do that, you'd still have the automated microphone, but you could of course plot. All you'd need to do is get the sound pressure levels and then plot them out in colored contours on a computer. So you can get these three-dimensional fields. Well, if you get these three-dimensional fields, it's fascinating to put the jug in the room and see how they change. They really chain significantly.

The first encounter I ever had with this was in '68, when we brought the first loudspeaker, a 901, to market. There was a person who was a New England conservatory graduate, a composer, and he worked for the company. And he really knew music. And he went to the show that used to be in New York, the consumer hi-fi show or whatever. And all of a sudden, I got a telephone call. He said, oh, my God. I don't know what's wrong, but the 901's sound horrible, and if we ever demonstrate this, we are dead. This is the introduction, and it just sounds terrible. And we changed them to another pair, and it's still the same. We changed the amplifier, it's still the same. This is a musician, now. So I had to take as one fact that it sounded terrible, because he knew it. And the rest-- he was changing amplifiers, he was changing everything.

Well, finally I asked him, how are you mounting the speakers? Where? The right distance from the wall, everything? Well, he was mounting them on a white box that had been constructed of very thin plywood. It was open on the bottom, but that was against the rug, and the box was about this big. Well, what was happening-- there's a compliance in the box and there's a mass of the plywood walls. And that was a resonance circuit. And so I said, go get a pedestal, quick, throw away the boxes, and call me back. See what happens. And the whole problem went away.

So people in their homes don't know this, and they can spend a lot of money for something and set it on an acoustical resonator, and it will sound terrible, no matter what the product is. So you have to watch out for physical structures. They really make a difference in the acoustics in a room.

Again, remember, on the first day, I mentioned about Professor Chu's way of looking at things. You see all the things that we've just been talking about-- we didn't solve three-dimensional acoustics specific to the room and the jug and all, but we have some basic knowledge now, that if the wavelength is large, pressures don't change from here to here. If the wavelength is 20 feet, I won't expect that the pressure is going to change much, or the velocity for example, when I move this much. So therefore, if the pressure were set to be 0 here, it's going to be 0 for an area, or going to be very small for an area. So just from one-dimensional analysis, you can already see how much you can extend your knowledge to situations that are beyond that.

OK. Now I forgot what I was going to do. Oh, acoustic circuits. All right. We already did one. There's something in acoustics that was one of the most early devices. This is the driving point here. I'll use this to indicate a source, like a piston pushing it back and forth. It could be a loudspeaker or anything. This was one of the early ones derived, and it's called a Helmholtz resonator. Again, whenever we're terminating, this will happen, by the way, unless we specifically give you a problem to ask if the termination on a tube is such a that it's valid to make the assumption that the elements are in series. Unless we ask a specific problem like that, we will always assume for the purposes of the subject, that they are, that this isn't a closed tube on an open tube.

OK let's say this is the area, A, of this cross-section tube here. This might be A2. We'll call this A1 and A2, L1, L2, L3. And we want to know what's going to happen out here. In particular, maybe we want to know the volume velocity that's coming out of here. VV2, I'll call it. So this is called, as I said, a Helmholtz resonator. So if we looked at that structure two weeks ago and said to find the volume velocity out of here, it'd be pretty formidable. Now it should be pretty simple. So let's make a circuit for this, and maybe we can look at the circuit and tell some things just from looking.

So here are the input terminals. Let's start. What do you want to connect across here?

SPEAKER 10: [INAUDIBLE] parallel [INAUDIBLE].

DR. AMAR G. BOSE: Inductance to ground? Or to--

SPEAKER 10: [INAUDIBLE].

DR. AMAR G. BOSE: This way. OK. Value rho 0 L1 over A1.

SPEAKER 10: [INAUDIBLE].

DR. AMAR G. BOSE: For this fellow here? Going down.

SPEAKER 10: Yeah, an inductor.

DR. AMAR G. BOSE: An inductor, rho 0 L3 over A-- I made a bad choice of variables-- L3 and A2.

SPEAKER 10: Going to a capacitor of V0 over c squared.

DR. AMAR G. BOSE: V0 over rho 0 c squared.

SPEAKER 10: And there should be another [INAUDIBLE] another conductor which represents L2 [INAUDIBLE].

DR. AMAR G. BOSE: Rho 0 L2 over A1. This is right on. How many of you followed that? OK. That's great, as far as I'm concerned, to be able to go that far from where we started today.

Now you mentioned that you didn't know where this one went. OK, let's think about it. What's the across variable here? Holy smokes. We all agreed that we liked the circuit, and we don't know what the across variable is. Pressure. Therefore, the through variable is volume velocity. Now, what's the assumption that we normally make going out here? Open tube. The pressure is 0 at this point. If the pressure, which is the across variable, is 0 at this point, where does this thing go? VV2. So here we now have a circuit in which the pressure is like voltage. Volume velocity is like current. And we need the transfer function from here to here.

Well, there's some things we can see about it right away. At the frequency in which this is resonant, what's the impedance seen looking from here to here? 0. Series resonance? The impedance of this is equal and opposite to the impedance of this. They're both reactive, but they have opposite signs. So there's a short circuit across here at that frequency. So guess how much comes out here, how much current flows down here when you have a short circuit across here and the series impedance? Not very much. So we know right away that whatever the frequency response looks like, this thing is going down to 0 at some omega 0, where omega 0 is the resonance of this thing here. Omega 0.

At DC, what's the transfer function from P to VV?

SPEAKER 11: 1?

DR. AMAR G. BOSE: 1?

SPEAKER 11: [INAUDIBLE] series inductors [INAUDIBLE].

DR. AMAR G. BOSE: So the transfer impedance now. I want VV2 over P at DC.

SPEAKER 12: [INAUDIBLE]. For one thing, all your assumptions are based on [INAUDIBLE] and for another, there's no way to generate [INAUDIBLE].

DR. AMAR G. BOSE: Yeah. This is like a short circuit across. This is exactly the same question as if I give you this. The voltage is here, just a simple inductor. The current is here. What's the current at DC? It's infinite, if this were an ideal inductor. If these aren't ideal and there's any loss, it's of course going to come way down. So what it does is we know it goes up to some level, but whatever the losses are or whatever's in there, as you say, there's a real problem when you get the DC. Are you adiabatic? The whole thing begins to fall apart. But we know it rises.

As omega goes to infinity, where do you think this thing goes? As you go to infinity, what does this element do?

SPEAKER 13: [INAUDIBLE].

DR. AMAR G. BOSE: Short circuit. 1 over j omega. So he goes to a short circuit. So I now have left a voltage divider of inductors to give the current here, for whatever pressure I put across here. So we know that this model, now-- not the physical device, the model-- then goes to some finite value, which, if you figured out the current that was coming in here, the product of this over this, the product over the sum would be the current going down here. You can all solve for that. So that comes to some finite value. So the thing looks like a resonance that has a frequency response curve like that.

Now let me take a little bit more complex one. And if you can get this level, you're well on your way to analyzing the practical things in acoustic circuits that you will come up against. OK. Let's take one, which is a screen in here, a screen in there, a pot, a big thing here, V0. Let's see. This whole thing, you could think this part as being circular if you want. Or it doesn't matter. These are big. Volume 1, volume 2. L2, I'll call this little length-- this I'll call R1, R2. All right. And let's call this distance-- I haven't used L1. Just to make it easy, I'm going to call cross section area of all this stuff the same. L2 was used, so this is L3, L4, L5. So here's the driving point. Whatever we're doing, driving that point. And here's the output. We want to make the equivalent circuit for that.